1. Find the vector of the form (0, 2) + λ(1, 1) with smallest L2 norm.

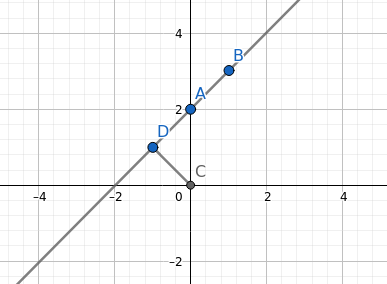
    2. A.:

    Solution 1:

    (0, 2) + λ(1, 1) = (λ, 2 + λ)

    ||(λ, 2 + λ)||22 = λ2 + (2 + λ)2 = 2λ2 + 4λ + 4

    Derivative: 4λ + 4 = 0, hence λ = − 1

    For λ = − 1, (λ, 2 + λ) = (−1, 1)

    Solution 2:

    geometric solution

    Point A is (0, 2). Point B is A + (1, 1). These two points determine the straight line parametrizes by λ in the statement. The L2 norm means "distance to the origin (0, 0)". The point in the line closest to the origin is D, with coordinates (−1, 1).